Mathematics Undergraduate Student Learning Objectives

The Mathematics program promotes mathematical skills and knowledge for their intrinsic beauty, effectiveness in developing proficiency in analytical reasoning, and utility in modeling and solving real world problems. To responsibly live within and participate in the transformation of a rapidly changing, complex, and interdependent society, students must develop and unceasingly exercise their analytical abilities. Students who have learned to logically question assertions, recognize patterns, and distinguish the essential and irrelevant aspects of problems can think deeply and precisely, nurture the products of their imagination to fruition in reality, and share their ideas and insights while seeking and benefiting from the knowledge and insights of others.

Students majoring in Mathematics attain proficiency in

Critical thinking

     The ability to identify, reflect upon, evaluate, integrate, and apply different types of in- formation and knowledge to form independent judgments. Analytical and logical thinking and the habit of drawing conclusions based on quantitative information.

Problem solving

     The ability to assess and interpret complex situations, choose among several potentially appropriate mathematical methods of solution, persist in the face of difficulty, and present full and cogent solutions that include appropriate justification for their reasoning.

Effective communication

     The ability to communicate and interact effectively with different audiences, developing their ability to collaborate intellectually and creatively in diverse contexts, and to appre- ciate ambiguity and nuance, while emphasizing the importance of clarity and precision in communication and reasoning.

     Students acquire and enhance these abilities in mathematical contexts, but the acquired habits of rigorous thought and creative problem-solving are invaluable in all aspects of life. These skills are acquired through experience in the context of studying specific mathematical topics and exploring problems chosen to challenge students’ abilities, spurring them on to acquire new techniques and abandon familiar but restrictive habits of thought. The overarching objectives can be realized in terms of more focused, appraisable objectives specific to mathematics as follows:

Critical thinking

     Students will

          • understand the basic rules of logic, including the role of axioms or assumptions

          • appreciate the role of mathematical proof in formal deductive reasoning

          • be able to distinguish a coherent argument from a fallacious one, both in mathematical reasoning and in everyday life

          • understand and be able to articulate the differences between inductive and deductive reasoning

          • proficiently construct logical arguments and rigorous proofs

          • formulate conjectures by abstracting general principles from examples.

     Courses: 20AB, 100, 101, 105AB, 110, 111AB, 117, 118, 160, 161.

Problem solving

     Students will be able to

• formulate and solve abstract mathematical problems
• recognize real-world problems that are amenable to mathematical analysis, and formulate mathematical models of such problems
• apply mathematical methodologies to open-ended real-world problems
• recognize connections between different branches of mathematics
• recognize and appreciate the connections between theory and applications.

    Courses: 19AB, 20AB, 22, 23AB, 24, 100, 101, 103AB, 106, 107, 114, 115, 116, 134, 145.

Effective communication

     Students will be able to

• present mathematics clearly and precisely to an audience of peers and faculty
• appreciate the role of mathematical proof as a means of conveying mathematical knowledge
• understand the differences between proofs and other less formal arguments
• make vague ideas precise by formulating them in mathematical language
• describe mathematical ideas from multiple perspectives
• explain fundamental mathematical concepts or analyses of real-world problems to non-mathematicians.

   Courses: 100, 101, 105AB, 111AB, 188, 189, 194, 195.

Subject-specific knowledge

     Students must demonstrate mastery in the three basic areas of mathematics: algebra, analysis, and topology/geometry on a basic level in lower division courses and at an advanced level in upper division courses.

Algebra, number theory, and combinatorics

     "we must endeavor to persuade those who are prescribe to be the principal men of our State to go and learn arithmetic, not as amateurs, but they must carry on the study until they see the nature of numbers with the mind only; not like merchants or retail-traders, with a view to buying or selling, but for the sake of their military applications and the benefit of the soul. . . I must add how charming the science is, and in how many ways it conduces to our desired end, if pursued in the spirit of a philosopher, and not of a shopkeeper!"

-Plato, The Republic

 

 

     Abstract algebra involves the study of algebraic structures such as groups, rings, fields, modules, vector spaces, and algebras. Linear algebra is a crucial subfield of algebra, both as an introduction to abstract algebraic structures and as a body of advanced results of immense importance in diverse areas of application. Number theorists study properties of the integers, as well as those of mathematical objects constructed from or generalizing the integers. Combinatorics involves finite or countable discrete structures, such as abstract graphs.

    Courses: Math 21, 100, 110, 111AB, 114, 115, 116, 117, 118, 120, 134.

Calculus and analysis

            Although the nature of,  … is in no way altered when they appear. . . on the right-hand side of the differential equation, nonetheless their role and the character of the equation are thereby altered. . . They are brought into the world unilaterally, shadow figures lacking the body which cast them ...The initiative is thus shifted from the right-hand pole, the algebraic, to the left-hand one, the symbolic.

Karl Marx, On the Differential

     Analysis extends and refines calculus; it encompasses differentiation, integration, measure, limits, infinite series, and analytic functions, primarily in the context of real and complex number systems. In much of analysis, the emphasis is not on finding explicit solutions to specific problems, but rather on determining which problems can be solved and what general properties solutions may share. Ordinary and partial differential equations play a central role in analysis, and are widely used in modeling real-world systems.

   Courses: Math 19AB, 20AB, 23AB, 24, 100, 103AB, 105AB, 106, 107, 140, 145, 148.

Geometry and topology

     "My noble friend, geometry will draw the soul towards truth, and create the spirit of philosophy . . . nothing should be more sternly laid down than that the inhabitants of your fair city should by all means learn geometry. Moreover, the science has significant indirect effects...in all departments of knowledge, as experience proves, any one who has studied geometry enjoys infinitely quicker understanding than one who has not."

-Plato, The Republic

     Geometry explores the implementation and far-reaching consequences of systems of mea- surement; topology addresses questions pertaining to shape and global structure. Non- Euclidean geometry, differential geometry (the extension of calculus to mapping of curves, surfaces, and their generalizations), and algebraic geometry generalize key results and tech- niques from Euclidean geometry to both familiar and exotic settings. In algebraic and differ- ential topology, techniques from diverse areas of mathematics are used to infer information about the shapes and related properties of spaces.

   Courses: Math 23AB, 121AB, 124, 128AB, 129, 130.

     This subdivision of mathematics is not sharp: the areas of overlap between the three main areas of mathematics are of great interest and importance. For example, analytic number theory as the study of the integers by means of tools from real and complex analysis, while differential geometry focuses on the interplay between analysis and geometry. The importance of all three areas, the influence of each on the others, and the insight to be gained by considering one area from perspectives commonly associated to another one are all emphasized in the Mathematics curriculum—as can be seen in the course matrix, many courses involve material from multiple areas.

The Mathematics Department offers three tracks within the Mathematics major:

Pure Mathematics

     Students in the Pure Mathematics track often go on to graduate study in mathematics; the pathway emphasizes the importance of a well-rounded, in-depth mathematical education, and includes advanced coursework in algebra, analysis, and geometry.

Computational Mathematics

     Students in the Computational Mathematics track explore applications of mathematics in other fields and gain experience in mathematical modeling of real-world phenomena using ordinary and partial differential equations, approximation and optimization techniques, linear programming, or game theory.

Mathematics Education

     Students in the Mathematics Education track prepare for a career in K–12 mathematics education; students acquire in-depth knowledge of subjects covered at an introductory level in the classroom, including number theory, classical geometry, and the history of mathematics, and gain experience in teaching mathematics in an accessible and intuitive, but precise, manner.

Curriculum matrix

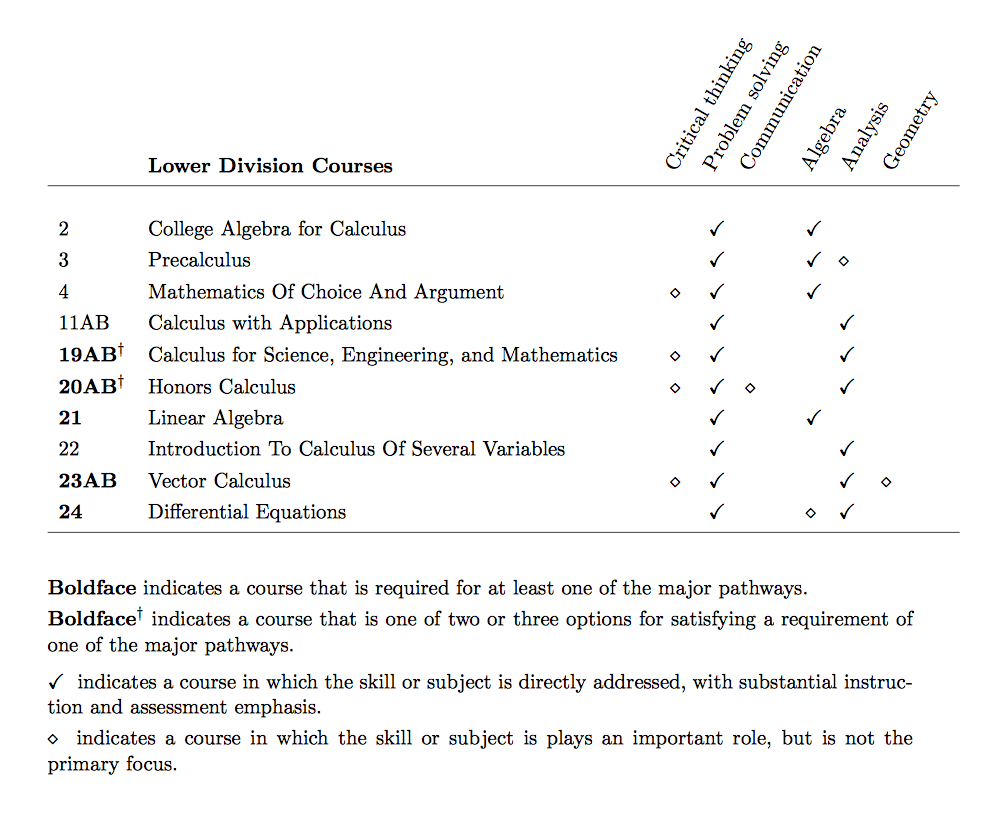
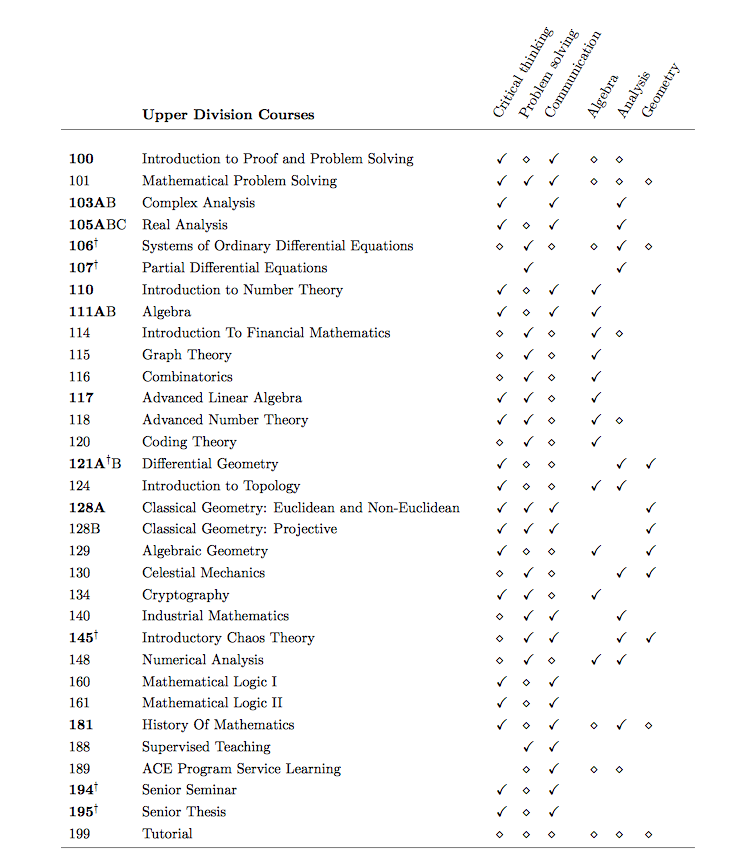
     All of the key objectives are addressed to some extent in all courses. For example, the ability to formulate precise mathematical statements and to reason logically are essential skills that are progressively developed throughout the curriculum. However, some skills are more heavily emphasized and utilized in some courses than in others. Some courses are specifically intended to help students move to a new level of proficiency with a particular portfolio of skills, while others are accessible only to students who have already reached a given level; the latter courses make heavy use of particular skills, and thus enhance and reinforce the student’s mastery of it, but the skills themselves are not the primary focus of such courses. Some connections between the key objectives, main subject-specific areas, and courses are indicated in the following tables of lower and upper division mathematics courses.