 Falling cats and stars in orbit embody mathematics in action for Richard Montgomery. Known for his engaging lectures to students and public audiences alike, Professor Montgomery uses the examples to convey the research that fascinates him.
Falling cats and stars in orbit embody mathematics in action for Richard Montgomery. Known for his engaging lectures to students and public audiences alike, Professor Montgomery uses the examples to convey the research that fascinates him.
A cat that jumps or gets dropped, as even children know, always lands on its feet. How does it manage this trick? The answer, as Montgomery points out on a poster in his office, is that the cat rotates its body around an axis by transforming its shape in midair.
This feline function sounds simple, but explaining how it works requires a branch of mathematics called gauge theory. The field captivated Montgomery as a student. "These are the deepest levels of mathematics, and they connect to problems in physics that interested me," he says.
 From R. Montgomery, 2001, "A new solution to the three-body problem", Notices of the American Mathematical Society, 48 (5), 471-481, 2001.
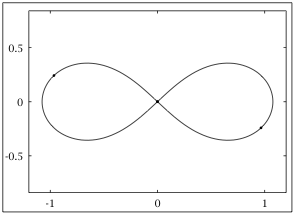
From R. Montgomery, 2001, "A new solution to the three-body problem", Notices of the American Mathematical Society, 48 (5), 471-481, 2001.
Several years after Montgomery came to UCSC in 1990, a colleague contacted him about an issue requiring a related analytical approach: the so-called "three-body problem." The problem boils down to this: If there is nothing else in the universe, what are all possible motions for three massive bodies? Again, a simple-sounding proposition is extraordinarily complex in detail.
"I remember thinking I didn't want to touch the three-body problem with a ten-foot pole," Montgomery recalls. "So many people had worked on it for 300 years. It goes all the way back to [Isaac] Newton." Still, the challenge was a powerful draw.
After what he calls "minor results," Montgomery started to work with Alain Chenciner at the Bureau des Longitudes in Paris. Within two years, they earned worldwide attention with a startling new solution.
In the orbit they discovered, three equal-mass stars or planets chase each other along a perfect figure-eight path. To the amazement of those who had worked on the problem for years, the figure-eight orbit proved stable. "It has a chance of existing in the universe, but the probability is extremely low," Montgomery says, noting that all three bodies must have masses within one-tenth of 1 percent of each other. "The importance is more in the techniques we pioneered."
Montgomery has collaborated with colleagues who apply similar methods to real-world systems, such as helicopters in flight or "tethered" satellites, to generate power in orbit. However, he prefers to develop the mathematical foundation for an ever-deeper understanding and, perhaps, for future flashes of discovery. "I compare it to going down a river no one has seen and figuring out how to get around the next bend," says Montgomery, an avid whitewater kayaker. "Mathematics is like that, but the exploration takes years instead of hours."
